Stupid physicists, they are doomed. Spending their whole lives searching for a theory of everything, not knowing that some eighty years ago this was proven to be logically impossible. 

The internet is full with sentiments like the above. Many such posts refer to Stephen Hawking's 2002 Dirac lecture Gödel and the End of Physics.

With the publication of his new book 'The Grand Design', the Oracle of Cambridge is again adding to the confusion. However, due to all the fuzz surrounding a much less interesting claim by Hawking this issue has not received any attention whatsoever.

What is Hawking telling us? In his Dirac talk he states:

"Up to now, most people have implicitly assumed that there is an ultimate theory, that we will eventually discover. Indeed, I myself have suggested we might find it quite soon. However, M-theory has made me wonder if this is true."

He continuous:

"Maybe it is not possible to formulate the theory of the universe in a finite number of statements. This is very reminiscent of Gödel's theorem. This says that any finite system of axyoms, is not sufficient to prove every result in mathematics."

and at the end of his talk concludes with:

"Some people will be very disappointed if there is not an ultimate theory, that can be formulated as a finite number of principles. I used to belong to that camp, but I have changed my mind."



Hawking, terminator of the TOE? Hawking's latest book must puzzle those who hailed his claim that TOE is dead.

Clearly, the world's most celebrated Nobel-less physicist is giving the physics community a wake-up call: "guys, you may not have noticed it, but we have hit a brick wall in our search for understanding and knowledge. Stop the search for the ultimate theory!". Since 2002 Hawking has delivered his talk and this very message several times, and a multitude of copies of the text of his talk can be found on the internet. So why are hordes of physicists stubbornly persisting in their doomed quest?

And even more amazingly: how on earth can Hawking himself end his most recent book with the very conclusion:

"M-theory is the only candidate for a complete theory of the universe. [..] If the theory is confirmed by observation, [..] We will have found the grand design"

Has Hawking again changed his mind? 

No, he hasn't. But he does seem to have gotten himself in a mess. Stuck in a labyrinth of excessive claims out of which no escape is possible. Life ain't easy if your books sell by the million and publishers insist on one-liners that guarantee a media frenzy.*

So let me try to help out here and give you my take on what Hawking is trying to convey. It all starts with this guy Gödel that Hawking refers to in his Dirac talk. 

From God To Gödel

Brilliant, perfectionist, obsessed and socially inept. Kurt Gödel was all of it to the extreme. A lively account of Gödel's social interactions with government officials as well as with his friend Albert Einstein, is given in a note from Oskar Morgenstern (a scan of the original can be found here). Gödel's friendship with Princeton Institute colleague Einstein lasted till Einsteins death. They both admired each other, and late in his life Einstein commented that "his own work no longer meant much, that he came to the Institute merely [..] to have the privilege of walking home with Gödel".



The privilege of walking home with Gödel

Both friends were titans of twentieth century science. Although Einstein is much better known to the wider public, without any exaggeration Kurt Gödel can be named the Einstein of logic. His famous 1931 article in the Monatshefte für Mathematik und Physik redefined the foundation of mathematical logic, and put clear limitations to what can be achieved by the methods of logic.   

Gödel's article presents a clever meta-mathematical argument that, put in simple terms, proves there is no system of logic that is free from contradictions and at the same time free of gaps. In other words, there is no system of logic that is capable to separate all statements contained within that system in the two categories 'true' and 'false'. There is always a third category 'undecideable'.

This incompleteness theorem** exploded as a bombshell and shook the whole world of mathematics. Eighty years later Gödels results continue to stand out as a key achievement that has forever redefined mathematical logic and continuous to influence its development.
 
Gödel's brilliance is beyond any doubt, but his mind also played tricks on him. Gödel's perfectionism created obsessions that in his later years grew far beyond his control. He stopped publishing his work out of fear for imperfections. Gödel's paranoia thereby deprived the world of any further contributions of this great mind. Other obsessions, however, affected Gödel himself. One of these was a fear of getting poisoned. Gödel trusted only the food prepared by his wife. When in 1977 she got hospitalized for six months, Gödel refused to eat. Late December 1977 the giant of mathematics was admitted to Princeton Hospital, weighing no more than 65 pounds. He died two weeks later, with the diagnosis "malnutrition and inanition due to personality disturbance".

Some forty years after Gödel's death, we are asking ourselves the question what the legacy of this man means for physics. 

Present day physicists feel little limitations. Frankly, they are the least impressed by any statements that try to limit their future achievements. This has been very different. Galileo's insights got overruled by biblical statements that declared the earth to be immoveable. However, that time now lays far behind us. The concept of separation of church and state has given physicists free reign. Since Laplace replied "Sire, I had no need of that hypothesis" to Napoleon's questioning why 'the author of the universe' was missing from his theories, no church and no God seem capable to stop physicists making progress. Almighty or not, physicists don't allow any supreme being blocking the road to new horizons and further progress.

Enter Gödel. Is he God's revenge? Is Gödel putting limits to what physicist can achieve?

Physicists build theories that are based on mathematics. They use mathematics to derive conclusions from these theories. They make predictions about physical reality based solely on mathematics and logic. Surely Gödel's results put limitations on what can be achieved in theoretical physics!

Wow, wow. Not so quick! 

We will come back to the question what is the relevance of Gödel to physics. But let us first make sure we understand Gödel's incompleteness theorem and its impact on mathematics. 

Gödel: God's Gift To Math

Fueled by inaccurate popularizations, the wider public seems to hold erroneous views on what Gödel's results imply. Following the adage a picture tells more than a thousand words, let me try to visualize how the vast majority of the public seems to perceive Gödel's results: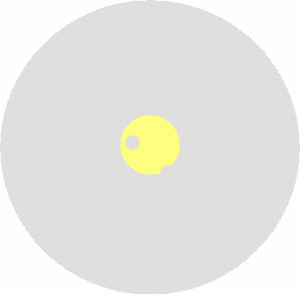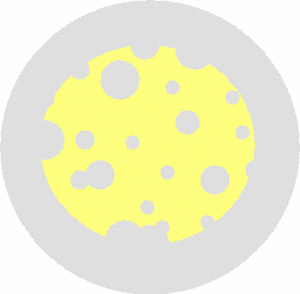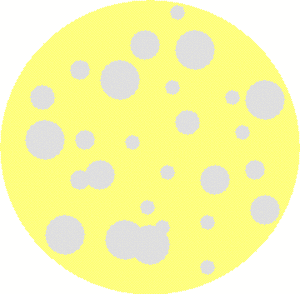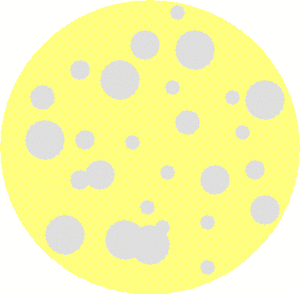



Visualisation of a popular myth of Gödel's impact on mathematics. In the space of mathematical propositions there are grey areas, statements that are not yet proven neither disproven. Mathematicians work hard to find proofs or disproofs for all statements, thereby reducing the grey areas. Gödel's results tell us that there will always remain grey areas.   
     
The above picture is misleading as it focusses on one single system of axioms. Mathematicians are not bound to work in a single such system, and are free to construct novel and more powerful systems. It is a freedom that is almost a defining characteristics of mathematics. A more accurate picture is therefore the following:



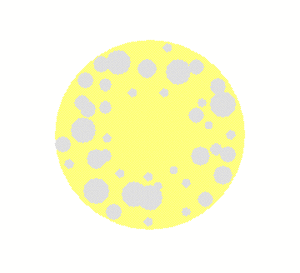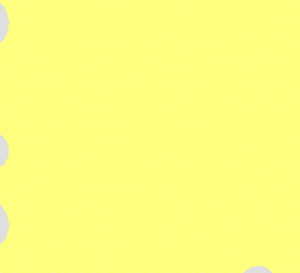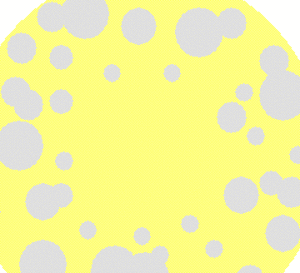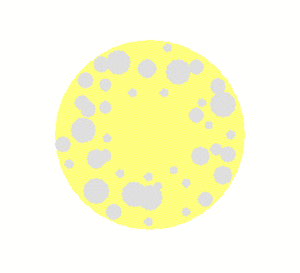
Improved visualisation of the impact of Gödel's results on mathematics. Each system of axioms leads to grey areas of propositions that are undecideable. Progress can be made by replacing the set of propositions by more powerful ones that have a wider range of application. This removes grey areas but at the same time opens up new horizons behind which new grey areas loom. This process can repeat endlessly.

This improved picture gives a much more optimistic view on the impact of Gödel's work on fundamental mathematics: there is no end to mathematics, progress is always possible but requires new approaches. Gödel has given mathematicians the guarantee they will never be out of work!

Since Gödel's 1931 publication, further work has elucidated the meaning of his incompleteness theorems. In particular, in 1936 Alan Turing*** gave a specific example of uncomputability: a problem that can not be answered by any computer no matter how powerful or how long one is prepared to wait. This uncomputability implies Gödel's incompleteness: the grey areas in above animations.

Uncomputability is a notion that brings us closer to physics. A theory of the universe is nothing more ( and nothing less) than a computer program. A program that simulates the evolution of the universe starting from the moment of the big bang. Gödel's and Turing's results make it very doubtful uncomputability issues would not await a theoretical physicist who has managed to construct the ultimate computer program that will simulate the universe.

So there you have it: Turing has made incompleteness issues entering physics.

Let's investigate this closer. 

From Gödel To Gravity

Turing's uncomputability refers to the generic problem of deciding whether any given computer program with given inputs will stop. Turing demonstrated that there exist computer program's with given inputs that can not be predicted to ever halt. You simply have to start the program and wait. As long as it keeps running you have no clue if it ever will halt.

So let's say we have a computer program that simulates the whole universe and that is started with inputs such that it will compute the universe of tomorrow. You start the giant computer that runs the program, and it starts cranking away. Huge amounts of data are processed. After an hour the computer is still running. Two more hours, still running. Twenty more hours, no sign the program will stop. Tomorrow passes by, and the computer is still running. Will it ever stop? You have no idea.  

So what? 

This basically shows that it is likely that physics will never be able to predict tomorrow in all its details. But we knew that already. Physicists can't predict a coin flip, let alone tomorrow's stock exchange prizes. So Gödel's incompleteness and Turing's uncomputability don't bring us any new results. 

But there is more. 

The above application of uncomputability to predicting the universe assumes we can in principle build a computer capable of simulating the universe. But we can not. Not even in principle. If you would attempt building such a computer, you would discover that it would collapse and form a black hole long before it reaches the size needed to be capable of simulating the universe. And this constitutes not just a practical problem, but a very fundamental limitation. Even if we would be capable of building a quantum computer out of smaller and lighter components, we would still hit the same problem. Make the compenents even less heavy until you hit the quantum limit and the Heisenberg uncertainty relations prevent you from further improving your computer. Even if you manage to built the ultimate quantum computer operating on the orchestrated motions of countless photons, all with zero mass, you would still witness black hole formation before you would reach the computational capacity that would alow you to predict tomorrow.

So it is gravity, and not Gödel, that prevents us predicting the future. That actually makes into a nice bumper sticker: "Gravity is to physics as Gödel is to math". This is the point Hawking is referring to in his Dirac lecture where he states "quantum gravity is essential to the argument".

But we are not done yet. 

As far as the question "is a TOE possible?" is concerned, all of the above is absolutely irrelevant. Uncompuability does not imply that a Theory Of Everything can not be constructed. Gödel does not prevent physicists from doing so, and neither does gravity. Stating that Godel (or Turing, or gravity) implies the logical impossibility of a TOE, is the same as stating that because of the incompleteness theorem an axiomatic logic can not be constructed. This is simply wrong. Axiomatic logics can be constructed, but given an axiomatic logic not every result can be derived. Similarly, Gödel nor gravity prevents us from constructing a TOE, but gravity does prevent us from turning this TOE into a crystal ball. 

Anyone a problem with that?

Notes

* It is a fun exercise to derive extreme headlines from the various 'Hawking one-liners'. My favorite one is derived from his most recent one-liner "God not needed!", combined with his book title "God created the integers", giving the headline: "Hawking: all of math wrong, integers don't exist!".
** Actually, Gödel derived two related theorems. I have not attempted to state precisely all relevant details and assumption behind Gödel's incompleteness theorems.
*** Another example of a life with a tragic ending. The UK of the post-war years was not particularly tolerant toward homosexuality. In the early 1950's, Turing's homosexuality resulted in a criminal prosecution and chemical castration. Shortly thereafter, aged 41, Turing committed suicide by cyanide poisoning. 

--------------------------------------
The Hammock Physicist on: E=m.c², Entropic Gravity, Entropic Force, Shut Down LHC?, Game Theory, Metric Vs Imperial, Big Bang, Dark Energy, Chaos And Time's Arrow, The Grand Arena, Square Root Of The Universe, Physics In A Nutshell, The Longest Path, Hotel Boltzmann, Quantum Telepathy, Quantum Viruses, QHD, Fibonacci Chaos, Counting A Black Hole, Entropic Everything