Although now widely accepted as the most natural explanation of the observed features of the universe around us, dark matter remains a highly mysterious entity to this day. There are literally dozens of possible candidates to explain its nature, wide-ranging in size from subnuclear particles all the way to primordial black holes and beyond. To particle physicists, it is of course natural to assume that dark matter IS a particle, which we have not detected yet. We have a hammer, and that looks like a nail.

Indeed the searches for dark matter at collider experiments have flourished in recent times, conforted by the fact that there exists a sort of "cosmic coincidence" between the abundance of a hypothetical weakly-interacting particle which is needed to explain the observed amount of dark matter in our universe and the abundance of such a particle that the big bang would have produced, IF the particle had a mass in the few hundred GeV range (and some assumed characteristics that well fit the classification as dark matter, such as the weak interaction with ordinary matter). And few hundred GeV are the bread and butter of LHC experiments these days!

A weakly-interacting particle in the few hundred GeV mass produced in abundance by the big bang and perfectly stable (such that it has remained around in the last 13.7 billion years) could well be the neutralino, the lightest particle predicted by Supersymmetric theories.

If Supersymmetry is the true extension of the standard model of particle physics, and if a quantity called "R-parity" is conserved (there are reasons to believe it should be; for instance, the fact that protons are very stable physical systems, as R-parity violation could produce proton decay), then the neutralino is a necessary consequence, and everything fits together quite nicely. But a heavy, weakly-interacting particle does not arise exclusively in SUSY theories, so one can search for it in more open-minded fashion. We can simply say that the LHC collisions could produce pairs of these bodies, which would then escape unseen without leaving energy deposits in the detectors; and examine the consequence of this.

A nice way to try and detect such a undetectable process is to rely on the fact that any energetic hadronic collision generates, in addition to the "hard subprocess" -say a quark-antiquark annihilation which produces a pair of dark matter particles- a thing called "initial state radiation". The colliding bodies in other words often lose some energy before they annihilate or scatter; the energy is sometimes emitted in the form of a single energetic photon. The photon can be detected, unlike the dark matter particles, so the event is not completely invisible any more!

The experimental signature involves observing an energetic photon recoiling against... nothing at all: the pair of dark matter particles carry away momentum and this betrays their escape. Events with a photon and so-called "missing transverse energy" (actually, missing transverse momentum, but the two things are experimentally the same thing) do arise from standard model processes but are quite rare, so that even a weak signal of dark matter pair-production can be detected as a surplus.

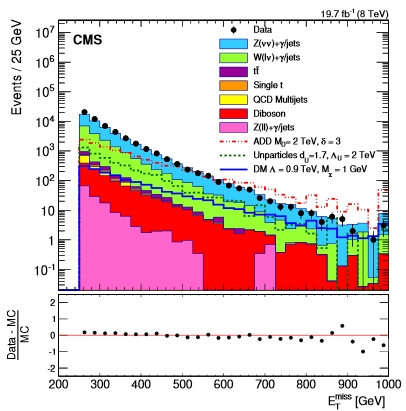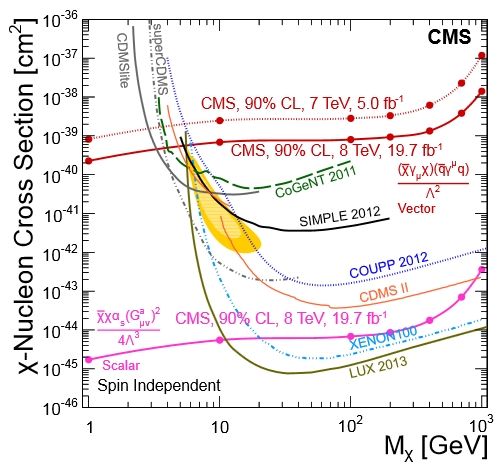
Have a look at the spectrum of missing transverse energy produced by CMS in 20 inverse femtobarns of proton-proton collision data at 8 TeV (see figure, right). The observed data (black points) follow very precisely the predictions of SM backgrounds up to very high values of missing energy, indicating that no signal of dark matter particles or similar entities can be hypothesized. In the graph isreported as an example a blue empty histogram which correspond to the contribution that a dark matter particle would yield to the data, for some specific values of its mass. (The graph also contains the hypothetical signal of unparticles and large extra dimensions, but those are different beasts and I won't discuss them here).

From the absence of a signal, CMS extracts upper limits on the cross section for interactions of dark matter particles with nucleons, as a function of the hypothetical dark matter mass. This is compared to other upper limits as determined by direct searches in the busy graph below. Note that the CMS limits from the search described here (marked "8 TeV" and shown as a red and pink line) depend on the kind of coupling of the dark matter to ordinary matter: a vector coupling would result in the weaker limit shown in red, while a scalar coupling would produce the much tighter limit shown in pink.



For more details of the CMS search please see the preprint here.