Last Transit of Venus was more fruitful than could be expected. Thousands of amazing images were taken and this is just enough to appreciate the high point of the event. Things more physical or astronomical were also concluded from it, of course.
One of these was the recalculation of the Astronomical Unit (au). As you may know, the astronomical unit is defined as the distance between the Sun and the Earth. Then the Sun is 1 au far away from the Earth. The nearest star to our Sun, the red dwarf star called "Proxima Centauri" is 268,000 au from the Sun. AU definition as the distance between the Sun and the Earth is fixed but, how can we measure that distance more accurately? Note that we do not know exactly what the value on one au is. Events such as the last transit of Venus will help to that task. First we have to remember some elemental Astronomy: the parallax.

What is the parallax?
It is simply an apparent shift relative to a distant background caused by a change in the position of the observer. Humans know what parallax is: look at a distant object with the left eye only and then do the same with the right eye. This change of position is parallax. The same occurs when we measure a distant start but now, since it is far, we may think that parallax does not occur but it always does, although it is not appreciated to the naked eye.
It seems easy to measure the distance between the Moon and the Earth. Since the Earth is moving around the Sun that measure is more tricky to calculate than may seem. Nearby starts seem to move with respect to the background sky. This apparent movement (only apparent) is called stellar parallax. Needless to say that parallax must be taken into account when calculating distances to far objects. Let us show this with an easy picture:

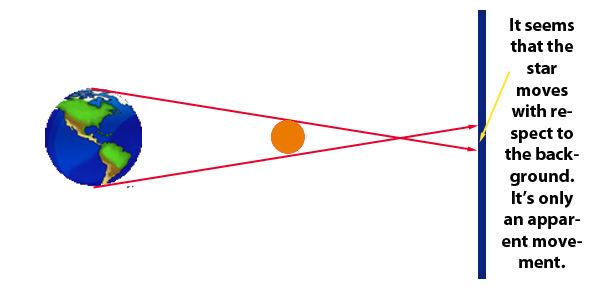
The measure of the distance to the star is different in the two places. Similarly, it is different if we measure it one month or several months later because the Earth revolves around the Sun. To fix this we make use of parallax. An easy trigonometry is needed:



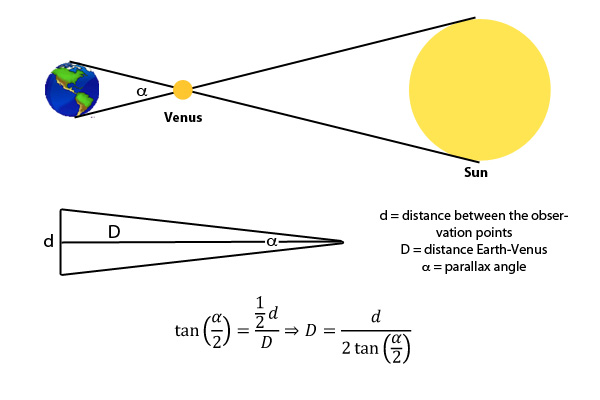
Note that in the upper triangle, the distance between the two observation points (d) must be taken in straight line, not on the curved Earth. The image shows how we can get the distant Earth-Venus (D) but we want to obtain the distant between the Earth and the Sun (to refine the Astronomical Unit). It is easy thanks to Kepler's Third Law which says: the square of the time a planet takes to go around the Sun equals to the cube of the distance of such a planet to the Sun. We know that Venus takes 0.6152 years to complete its orbital period, then the distant above is 0.7233. That is, the distance between Venus and the Earth is 0.2767 (au), so
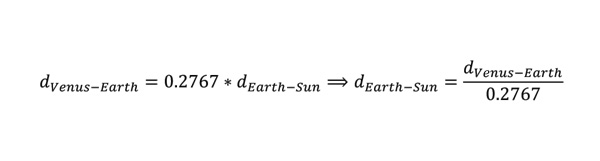
If we know the distance to Venus we can easily know that the distance to Sun is (that is, the value of 1 au).

How can we benefit of last Venus transit?
As reference images and with the aid of a telescope and a computer we can take four images to compute the value of the au:

1) The disc of Venus seems to touch the disc of the Sun in the first moment of the transit.
2) The disc of Venus seems to lose contact with the disc of the Sun in the first moment of the transit.
3) The disc of Venus seems to touch the disc of the Sun in the last moment of the transit.
4) The disc of Venus seems to lose contact with the disc of the Sun in the last moment of the transit.

These images allow us to calculate au value in a more accurate way. We will have to wait until astronomers provide the new recalculation of a unit whose current value is 149,597,870.7 km.