Michael Lewis's bestselling book "Flash Boys" describes how some brokers, engaging in high frequency trading, exploit fast telecommunications to gain fraction-of-a-second advantage in the buying and selling of stocks. But you don't need to have billions of dollars riding on this-second securities transactions to appreciate the importance of fast signal processing. From internet to video streaming, we want things fast.

Paul Lett and his colleagues at the Joint Quantum Institute (1) specialize in producing modulated beams of light for encoding information. They haven't found a way to move data faster than c, the speed of light in a vacuum, but in a new experiment they have looked at how light traveling through so called "fast-light" materials does seem to advance faster than c, at least in one limited sense. They report their results (online as of 25 May 2014) in the journal Nature Photonics (2)

Seeing how light can be manipulated in this way requires a look at several key concepts, such as entanglement, mutual information, and anomalous dispersion. At the end we'll arrive at a forefront result.

CONTINUOUS VARIABLE ENTANGLEMENT

Much research at JQI is devoted to the processing of quantum information, information coded in the form of qubits. Qubits, in turn are tiny quantum systems---sometimes electrons trapped in a semiconductor, sometimes atoms or ions held in a trap---maintained in a superposition of states. The utility of qubits increases when two or more of them can be yoked into a larger quantum arrangement, a process called entanglement. Two entangled photons are not really sovereign particles but parts of a single quantum entity.

The basis of entanglement is often a discrete variable, such as electron spin (whose value can be up or down) or photon polarization (say, horizontal or vertical). The essence of entanglement is this: while the polarization of each photon is indeterminate until a measurement is made, once you measure the polarization of one of the pair of entangled photons, you automatically know the other photon's polarization too.

But the mode of entanglement can also be vested in a continuous variable. In Lett's lab, for instance, two whole light beams can be entangled. Here the operative variable is not polarization but phase (how far along in the cycle of the wave you are) or intensity (how many photons are in the beam). For a light beam, phase and intensity are not discrete (up or down) but continuous in variability.

QUANTUM MUTUAL INFORMATION

Biologists examining the un-seamed strands of DNA can (courtesy of the correlated nature of nucleic acid constituents) deduce the sequence of bases along one strand by examining the sequence of the other strand. So it is with entangled beams. A slight fluctuation of the instantaneous intensity of one beam (such fluctuations are inevitable because of the Heisenberg uncertainty principle) will be matched by a comparable fluctuation in the other beam.

Lett and his colleagues make entangled beams in a process called four-wave mixing. A laser beam (pump beam) enters a vapor-filled cell. Here two photons from the pump beam are converted into two daughter photons proceeding onwards with different energies and directions. These photons constitute beams in their own right, one called the probe beam, the other called the conjugate beam. Both of these beams are too weak to measure directly. Instead each beam enters a beam splitter (yellow disk in the drawing below) where its light can be combined with light from a local oscillator (which also serves as a phase reference). The ensuing interference patterns provide aggregate phase or intensity information for the two beams.

When the beam entanglement is perfect, the mutual correlation is 1. When studying the intensity fluctuations of one beam tells you nothing about those of the other beam, then the mutual correlation is 0.

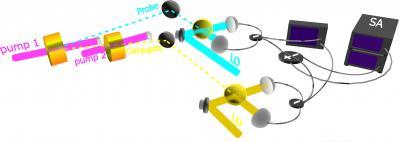
This image depicts the experimental setup for studying fast light. Pump beams (purple) create correlated probe (turquoise) and conjugate (gold) beams. Each of these beams is aimed at a beam splitter (yellow disks). A local oscillator (LO) also sends a laser beam into each of the beam splitters. The resulting interference pattern -- registered in a spectrum analyzer, SA -- for the probe and conjugate arms are compared.

(Photo Credit: NIST)

FAST-LIGHT MATERIAL

In a famous experiment, Isaac Newton showed how incoming sunlight split apart into a spectrum of colors when it passed through a prism. The degree of wavelength-dependent dispersion for a material that causes this splitting of colors is referred to as its index of refraction.

In most materials the index is larger than 1. For plain window glass, it is about 1.4; for water it is 1.33 for visible light, and gradually increases as the frequency of the light goes up. At much higher frequency (equivalent to shorter wavelength), though, the index can change its value abruptly and go down. For glass, that occurs at ultraviolet wavelengths so you don't ordinarily see this "anomalous dispersion" effect. In a warm vapor of rubidium atoms, however, (and especially when modified with laser light) the effect can occur at infrared wavelengths, and here is where the JQI experiment looks.

In the figure above notice that the conjugate beam is sent through a second cell, filled with rubidium vapor. Here the beam is subject to dispersion. The JQI experiment aims to study how the entanglement of this conjugate beam with the probe beam (subject to no dispersion) holds up.

When the refraction is "normal"---that is, when index of refraction causes ordinary dispersion---the light signal is slowed in comparison with the beam which doesn't undergo dispersion. For this set of conditions, the cell is referred to as a "slow-light" material. When, however, the frequency is just right, the conjugate beam will undergo anomalous dispersion. When the different frequency components that constitute a pulse or intensity fluctuation reformulate themselves as they emerge from the cell, they will now be just slightly ahead of a pulse that hadn't gone through the cell. (To make a proper measurement of delay one needs two entangled beams---beams whose fluctuations are related.)

CAUSALITY

No, the JQI researchers are not saying that any information is traveling faster than c. The figure above shows that the peak for the mutual information for the fast-light-material is indeed ahead of the comparable peaks for an unscattered beam or for a beam emerging from a slow-light material. It turns out that the cost of achieving anomalous dispersion at all has been that additional gain (amplification) is needed, and this amplification imposes noise onto the signal.

This inherent limitation in extracting useful information from an incoming light beam is even more pronounced with beams containing (on average) one or less-than-one photon. Such dilute beams are desirable in many quantum experiments where measurement control or the storage or delay of quantum information is important.

"We did these experiments not to try to violate causality, said Paul Lett, "but because we wanted to see the fundamental way that quantum noise "enforces" causality, and working near the limits of quantum noise also lets us examine the somewhat surprising differences between slow and fast light materials when it comes to the transport of information."

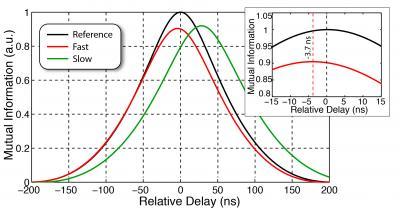
The mutual information of the two beams (how much we know about one beam if we know the fluctuation of the other beam) peaks at different times depending on whether the conjugate beam passes through a fast-light medium (red), a slow-light medium (green), or no medium at all (black).

(Photo Credit: NIST)