Perhaps what I really want to say is that what we study in particle accelerators may be tiny and ephemeral, but these quarks are real things. Quarkonium resonances are as real as kidney stones or speeding tickets, although unfortunately only the former can be expected to vanish in a flash of light before you can size them up.
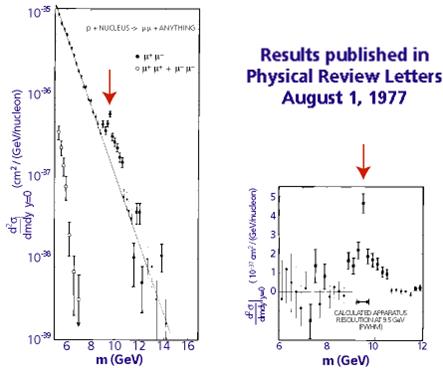The Upsilon particles have been discovered 35 years ago at Fermilab by a team led by Leon Lederman -a feat that eventually granted the American physicist a Nobel prize, after he had spent almost a decade wondering what the heck was that hump he had produced in the mass distribution of a similar lower-energy experiment, and three more years spent banging his head for not having been able to demonstrate it was the J/ψ meson (I already told the story of that "Lederman shoulder" elsewhere). Upsilon mesons produced in the collision of 400 GeV protons with a fixed target were observed to decay into pairs of energetic muons, producing a bump in an otherwise steeply falling background spectrum in the combined mass distribution of the muon pairs (see graph on the right; the left panel shows the mass distribution, the right panel shows the excess of data over the exponentially falling background of random muon pairs).Nowadays rather than banging nuclei with energetic protons the most effective way to produce Upsilon mesons is to collide electrons with positrons at a center of mass energy exactly equal to their rest mass. The BaBar and Belle experiments, in fact, are designed to exploit the large production rate of B mesons which get produced when the Y(4S) is created and decays.
The experimental facilities hosting these detectors are respectively SLAC at Stanford (USA) and KEK at Tsukuba (Japan), and they are quite similar in their general design (on the left, the Belle detector, courtesy Wikipedia). The collisions are actually made "asymmetric" by imparting more energy to one of the two projectiles, so that the center of mass of the pair moves in one direction, making the detection of final state products easier.
The above might have left you wondering why I mentioned the Y(4S) and what that thing really is. Indeed, there is a whole family of Upsilon states, labeled by their angular momentum with a notation which is still reminiscent of spectroscopy from over 100 years ago. The Y(1S) is the "fundamental state", and the lowest-mass one. At 9.46 GeV it is quite heavy but still its mass is insufficient to allow the decay to a pair of B mesons (each of the latter weighs 5.23 GeV). The same goes for the Y(2S) (10.02 GeV) and Y(3S) (10.36 GeV).
Only the Y(4S), at 10.58 GeV of mass, can decay to two B mesons, and so it does most of the time (in the orderly subatomic world, everything that is not forbidden is mandatory!), producing large samples of B mesons in Belle and BaBar. But these experiments also study the lower-energy states, as in the analysis which we are discussing today. To understand a bit more why what has been measured by Belle is important, let us make a digression here.
Imagine you're a Upsilon...
Now, it is fruitful to try and put ourselves in the place of a Y(1S) meson, to understand the difficult choices the thing has to make in order to eventually meet its destiny -the decay into lighter bodies. Only, to get into the script better, it makes sense for you to convince yourself that since you are a Upsilon, you want to decay -it's teleology, baby. Ready ? Let's go.
We get created as a planetary system of a bottom and an antibottom quark, each orbiting around the common center of mass, and tied to each other by exchanging a multitude of sticky gluons. We are heavy, so there is plenty of energy to release in the decay to lighter particles. However, let us actually examine our choices.
The first plan that crosses our Y mind is to let the bottom and the antibottom quarks in our belly annihilate, emitting gluons. Why -the bottom and antibottom quarks are one the antiparticle of the other, so it just requires them to get their wavefunctions to overlap in spacetime and puff...
But note that we first of all think of emitting gluons because gluons are much more readily emitted by a coloured quark line than photons (or god forbid, heavy and off-shell weak bosons -that would force us to wait for decades!).
We immediately realize we have a problem: the pair of quarks in our belly is globally colourless -we are colourless because they are. So the duo cannot annihilate into a single gluon -the gluon must carry colour! We therefore need two gluons emitted simultaneously (a system of N>1 gluons can be colourless overall!), which is a bit harder -less probable, so it takes more time to happen. But maybe not much ? We start to be tickled by the chance of rapidly decaying...
But no! Not even two gluons will do: two gluons together may be colourless, so we would not break any quantum chromodynamical rule, but they possess a negative value of the multiplicative quantum number called "C-parity", which is conserved by strong interactions. Now, the C-parity of the Y is also negative, so the process Y->gg cannot occur, because C in the initial state is negative, and positive in the final state (the two C-parities multiply, and minus times minus is plus).
Executive summary: Damn it! strong interactions will require at least three gluons, so that (-1)x(-1)x(-1)=-1, and the negative C-parity of the Y remains unaltered by the strong process! But this multiple gluon emission process is very rare (every other gluon you emit forces you to pay an α_s^2 factor, where α_s is the strong coupling constant, which is a number equal to about one fifth at the energy of the process), and it means that the decay takes a long time.
It seems we have discovered a subatomic conspiracy that prevents us from decaying quickly. Indeed, the above "selection rule" that demands that at least three gluons are emitted in the hadronic annihilation of a Y(1S) even has a name: it is called Okubo-Zweig-Iizuka (OZI) rule (NB the OZI rule is slightly different from what I have explained above, but the effect is the same as the one described). The same mechanism, by the way, is the one that makes the famous J/ψ meson also rather easily discernible in its decays to electron or muon pairs. But let's not divagate.
Let us instead examine other possibilities. Could we decay by strong interactions by creating two particles, one containing a bottom quark and a lighter antiquark, and the other containing the antibottom quark and a light quark ? This is a good plan: we "pick from the vacuum" a light quark-antiquark pair, stick each to one of the two b quarks, and we are done... Unfortunately, that does not work at all: the two B mesons we'd create would have a total mass far in excess of our total energy. We only weigh 9.46 GeV, while the two B mesons would weigh a total of 10.46 GeV. Alas, it's not so easy!
So we are left with the option of annihilating the b and the anti-b quark by emitting a photon; the photon can then materialize into anything viable, say a light quark-antiquark pair, or an electron-positron pair, or a muon-antimuon pair... This is of course possible, but electromagnetic interactions are sloooowwww. Is there anything else we've not considered yet ?
Well, yes and no. In principle, one of the two constituent b-quarks could decay by weak interaction into a charm quark, emitting a virtual W boson; the latter could then turn either into a quark-antiquark pair or a lepton-neutrino pair. Alas, this however is a weak interaction process: W bosons are very heavy -80 GeV their mass- and convincing one of them to fluctuate to an energy of just a few GeV, such that it can participate in the decay process of the b-quark into a charm, is a lenghty matter. W bosons do get that "virtual" from time to time, but if you ask a neutron how long it takes, you'd be surprised: the neutron decays to a proton, an electron, and an antineutrino in 900 seconds! A true eternity in subatomic standards!
All in all, we (the Y(1S)) are bound to decay either by emitting three gluons -which then materialize into several light hadrons- or by emitting one single photon. The rarity of the strong decay to three gluons make that decay slow enough that we have a chance to actually decay electromagnetically: the electromagnetic interaction is for once competitive with the strong one!
The Belle search
All the above is instructive but not too exciting: we have just learnt how a Y(1S) can decay to different final states, and the interaction which is responsible of the process. What I have not mentioned yet, however, is that the three-gluon decay cannot be directly seen: we see a bunch of hadrons coming out, but we usually cannot trace this to the explicit decay of a Y(1S).
That is (take a big breath): although we know that most of the time it has been a Y(1S) decay what produced the final state particles -that is because we can actually compute that a sizable fraction of the "at resonance" electron-positron collisions we generated has indeed created a Y-, only in a small fraction of the cases we can take the final state particles and say "you, you, and you together are the direct product of Y disintegration", such as in the reaction Y -> A+B+C.
The difficulty lies in the fact that these so-called "exclusive" hadronic processes (ones where we observe exactly all that is involved in the reaction) are relatively rare, and hard to detect. Much simpler is to detect the Y->μμ decay, but this occurs only 2.5% of the time (electromagnetic interactions compete with the three-gluon mode, but still take globally only a small fraction of the total possibilities). And thank goodness this number is still sizable, lest the Y would have been discovered with much more trouble! Again, we owe it to the Okubo-Zweig-Iizuka rule.
Now, the Belle analysis is complex and I am not going to describe it here, but rather point the interested reader to the preprint published two days ago by the Belle collaboration. Here, I am just going to list the reactions that have been observed (which, if it looks unintelligible to you, you can jolly well ignore),
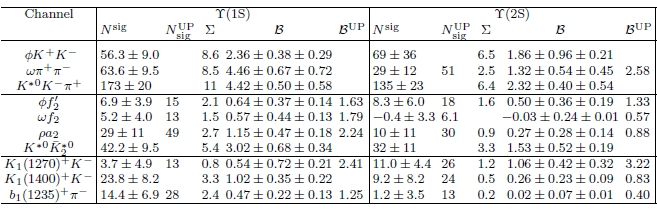
and offer a set of "signal plots" showing the partial resonances reconstructed from a subset of the charged particles identified in the final state: the data in the figures are shown by black markers with error bars, the red line is the total fit, and backgrounds are shown in grey and in dashed blue. The top row shows the φ meson signal reconstructed from kaon pairs (φ->KK), the middle row shows the ω signal from triplets of pions (as in ω->π^+ π^- π^0), and the bottom row shows the K*(892) signal reconstructed from its decay into Kaon-pion pairs (K*->Kπ). The panels on the left refer to the Y(1S) sample, the panels on the right refer to the Y(2S) sample.
The nice new result by Belle has been produced thanks to the huge samples of Y(1S) and Y(2S) events acquired by the detector in multi-inverse-femtobarn datasets of electron-positron collisions run at the energy corresponding to the mass of these resonances: over 100 million Y(1S) and over 150 million Y(2S) decays, plus an additional 89.4 inverse femtobarns of data taken off-resonance to study backgrounds. The Review of Particle Properties will need to add a few lines to the tablesof studied decays of these beautiful mesons: the list was already long, but most of the listed decays were partially-reconstructed ones, or just upper limits. So, kudos to Belle for a significant advance in the knowledge of quarkonium!
Comments