There are linear field equations of gravity called gravitomagnetism. It starts from the best starting place we have, general relativity. At the end of my talk last year at the 13th Eastern Gravity Meeting in Raleigh, someone asked me to compare gravitomagentism with my hypercomplex number gravity field equations. I knew they were different, but had not dived into that subject in detail. My talk this year at the 14th Eastern Gravity Meeting was a shop and compare between the two proposals. The blog will serve up the meat and potatoes of that talk at Princeton, Friday June 3, 2011, at 4:40pm.

In my title slide, I promoted Science20.com. I told the audience that if they found my work interesting (not necessarily right), they should visit here for a weekly dose of Doug. I also lamented that the weekly production rate is hard to maintain.
[Click or skip this reading of the blog]
Solutions to the non-linear general relativity field equations are hard to find. We focus our attention on the few that have been found decades ago. Working with those solutions is difficult. Much of the time in the two days of talks was devoted to struggles with numerical solutions that might provide signals for a gravity wave detector to parse.

Faced with such technical challenges, it might be easier to work with linear field equations. We live in a nearly flat Universe. Why not model such a Universe as being totally flat with a perturbation? That is what is done. Construct a wave equation for flat spacetime with a perturbation, choosing the transverse gauge because that makes the equation take the following simple form:

where h is the perturbation, and that funny Greek letter is the flat metric. This looks darn similar to the Maxwell equations written in the Lorentz gauge:

The big difference between these two is the rank of the equations. I don’t understand the details of this move, but the well-schooled talk about being only interested in the retarded solutions. This allows them to focus on a slice of the rank two equation:

Where one is using mass density and a matter current. This equation will be constrained to work for low density and slow moving stuff. With all these assumptions in place, one can write down four field equations:

where
When I read this, I got upset. Learning physics on my own has been a slow, hard climb. My studies have all centered around special relativity, the Maxwell equations, gravity, and quantum mechanics, ignoring all other fine subjects such as solid state physics or chaos theory. I learn by doing and making mistakes. These equations reminded me of mistakes I had already made in my efforts to construct field equations for gravity.


Take the big picture view: how are the four equations of gravitomagnetism different from the Maxwell equations? I know the factors of a half are tossed in to satisfy the spin 2 gods, but the gravitomagnetism field equations are clones of the Maxwell equations. I use the word clone with a negative spin. Such clones are evil because for gravity like charges attract while in EM, like charges repel.

I could spot four fatal flaws. The E and B fields can be written as an anti-symmetric rank 2 tensor. Changing the order of the indices flips the sign. That is the sign of a spin 1 field. A spin 1 field mediates like charges that repel. Bullet in the head 1.

To get the currents, there would need to be a vector current coupling term in the Lagrangian, a JuAu. If you spend some quality time with chapter 3 of “Feynman Lectures on Gravitation” as I have because of a humbling exchange with a physics professor, you know that a vector coupling term indicates a spin 1 field. Bullet in the head 2.

A metric is symmetric. Gravity is all about changes in metrics. A change in metric will also be symmetric. Changes in a symmetric metric cannot be accounted for by an anti-symmetric field strength tensor. Bullet in the head 3.

The no monopoles laws for gravity would mean that there could be two poles for a gravity source if it were put in motion. With two poles, one would attract, the other would repel. Two charges for gravity is not what we have ever seen. [Questionable] bullet in the head 4.

I was so upset by my initial study, I emailed the professor who wrote a brief review on the subject. He assured me that these problems were already known. That was a relief since it meant I had a good self-education. These fatal flaws are accepted because gravitomagnetism is useful. There are problems that would not be possible to think about without these simpler expressions. Professionals understand the limitations of this approach. This is a toy theory.

Toy theory, as a label, sounded too generous. I think it should be called a broken toy theory. I object to calling it a linear perturbation of general relativity. The label implies the equations result in a linear approximation of general relativity. A valid linearization will chop off the non-linear parts, but will not introduce new problems. Gravitoelectromagnetism introduces fatal flaws.

If something is broken, fix it now.

That is an attitude I learned at the bench. Nature doesn’t care if you get things wrong. She does not speak up if you continue down an errant path.

My correction involves forming a Lagrange density using hypercomplex products. A Lagrange density is every way energy can be exchanged per unit volume. Taking different derivatives ends up at the field equations or stress-energy tensor. Hypercomplex products sounds scary, but it is a simplification of familiar things if you have worked with complex numbers and vectors. Start with complex multiplication. Make the imaginary i a three vector I. Include a cross product. These are the rules of multiplication of quaternions: complex numbers and a cross product. To make the rules of multiplication for hypercomplex numbers, eliminate any minus sign. Like the Lagrangian for the Maxwell equations, there is a current coupling term and the derivatives of a potential. In other words, the same players take the stage but they do a different dance since the rules of multiplication have been altered. Here then is my hypercomplex gravity Lagrangian:

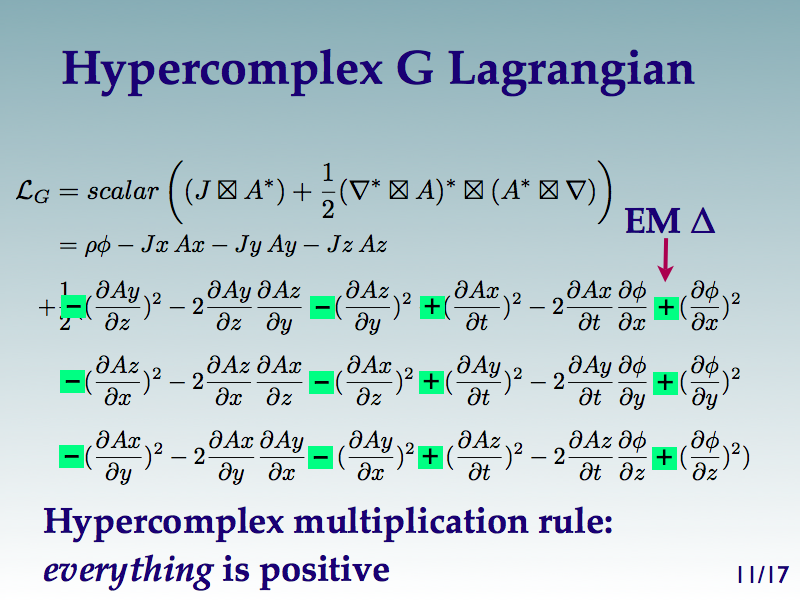
Every term that appears in the EM Lagrangian also appears for the hypercomplex gravity Lagrangian. The two cross terms keep their signs, but the other four flip signs. Generate the field equations the standard way by applying the Euler-Lagrange equations. To make Gauss’s law, take the derivatives with respect to phi:

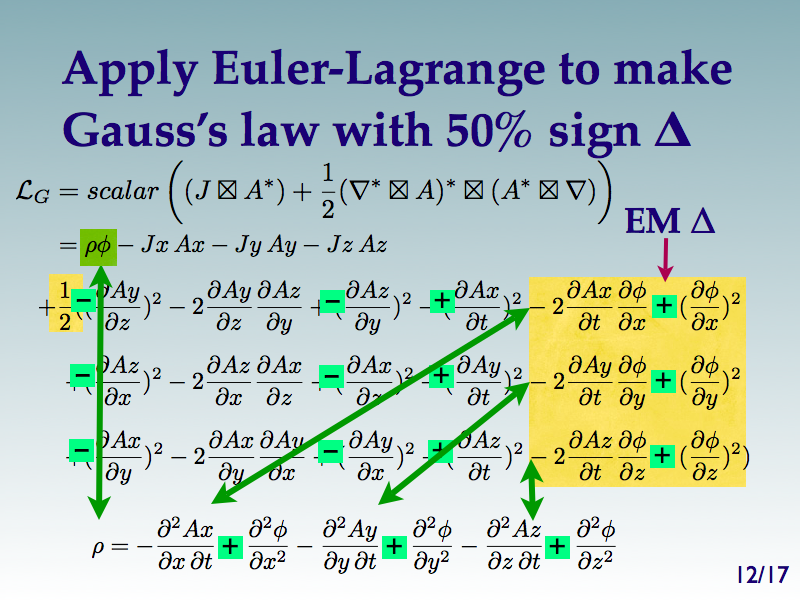
There is Gauss’s law, with 50% of the signs changing. Notice they are the ones needed for Newton’s law of gravity. Newton’s effort suffers from the problem that a change in the mass density would have to propagate instantaneously. These field equations do not have that flaw since there are time varying terms.

The same sort of exercise can be done to generate Ampere’s law. 


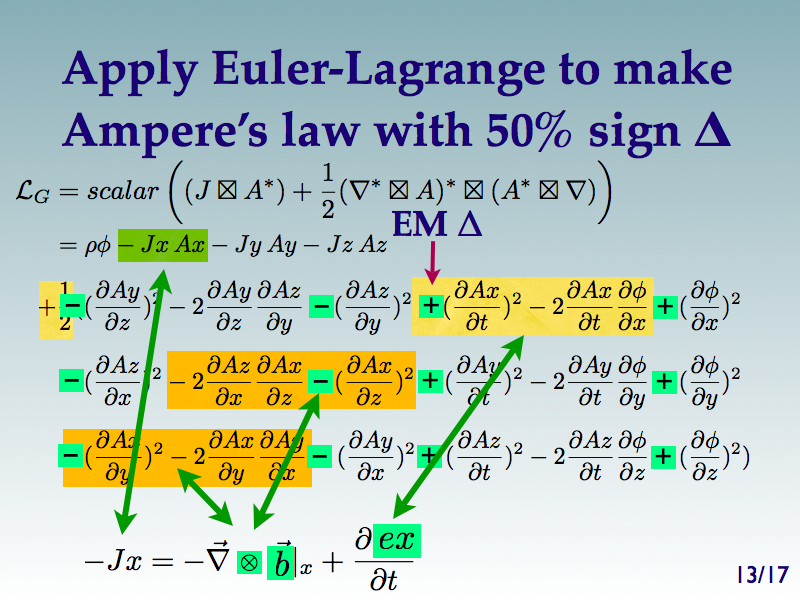
Here again, 50% of the signs change. That is important because it means that the Ampere’s law for EM is distinct from this proposal for gravity. Gravity has but one charge, so it should be distinct from EM which has two.

There is no hypercomplex gravity monopoles law or Faraday’s law. I consider that a good thing. Masses look like gravitational monopoles. Shake an electron up, and there are two poles so the magnetic field can both attract and repel. This is a relativistic reflection of the fact that there are two signs for electric charges. Shake up a mass, and there will be an effect, but there should only be attraction since there is only one type of mass charge.

After all this time thinking about this issue, I think I can pinpoint where the error happens. The linear perturbation of general relativity in equation 1 does have a spin 2 field. That can be seen because swapping the indices of the perturbation does not change the sign. It is only when they downshift to a rank 1 field theory that the problems are generated. After all, one goes from ten field equations to four. That has consequences. It is these four equations that are known as gravitomagnetism. I am at peace with the linear perturbation of general relativity, but not gravitomagnetism, the broken toy theory.

I posed the audience of about forty a question: How does this work affect you? I said my unified field equations predict the LHC will see no Higgs nor a single SUSY particle. Given the time limitation, I was not able to go into detail about not needing the Higgs as I did in an earlier blog.

If the LHC does find a Higgs boson (follow Tommaso for the latest), or one or more SUSY particles, then my work is wrong. Such a discovery will save me a $500 visit to the 15th Eastern Gravity Meeting in Syracuse. While it would be disappointing to be wrong, I would not feel so bad since unifying gravity with the standard model is a difficult task. Failing on a tough job is to be expected.

If the LHC does not find the Higgs boson or one of the SUSY particle like every other collider experiment before it, then the hypercomplex gravity and GEM unification proposal could be right. Here is my final slide:

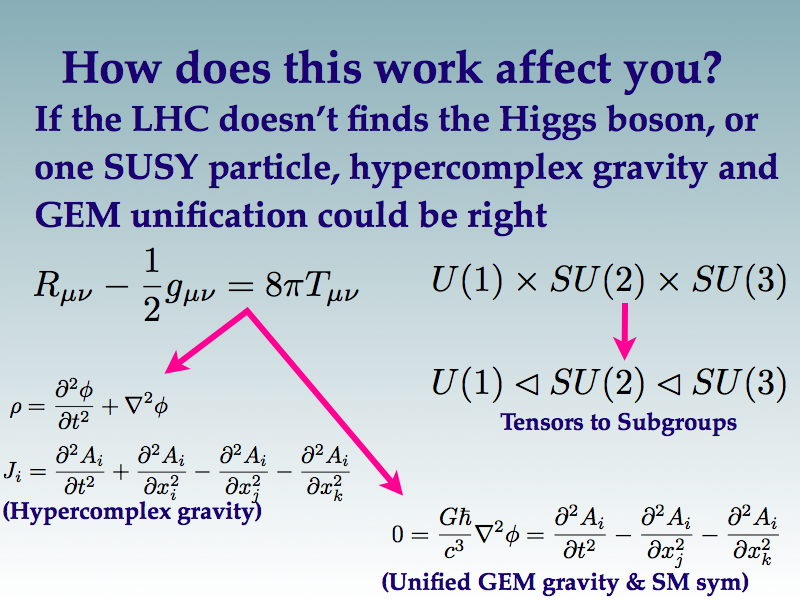
I pointed out three things about the GEM unified field equations. One was the lack of a unified current. In EM, like charges repel. For gravity, like charges attract. Since one cannot have a charge that both attracts and repels, when the two are united, there is no current density. To make the field equation dimensionless, I needed an area. I put in the Planck area which has units of relativistic c, quantum hbar gravity G. My goal was to make things dimensionless, and I end up with a relativistic quantum gravity expression Poisson would have been familiar with. The final observation was about the ability to choose a gauge. This is a vital issue at the Lagrange density level. Generate the field equations, then pick a convenient gauge for the problem at hand. That is how it works in EM and general relativity. With the GEM proposal, both the Lagrangian and resulting field equations are invariant under a gauge transformation. If anyone reading this wants to reflect on what it means to have field equations that are invariant under a gauge transformation, please speak up, I would like to hear.

We know the one electric charge is conserved. We know three weak charges are conserved. We know the eight strong charges are conserved. We associate the symmetries of U(1), SU(2), and SU(3) with each of those conserved charges respectively. It is natural to suggest that we therefore need the tensor product of these three symmetries. That means a Lie algebra with 12 elements. Based on discussion that happened here on Science20.com, my initial effort to recreate that tensor product with quaternions failed. I was force to admit I had a hybrid: a tensor product of U(1) and SU(2), but to look at SU(2) as a subgroup of SU(3). When I lost my electroweak symmetry, I shifted to the view of looking at U(1) which a subgroup of SU(2) which is a subgroup of SU(3). If one can write a Lagrangian with SU(3) symmetry, it necessarily has SU(2) and U(1) symmetry. That might be all one needs. That would have all of eight generators for its Lie algebra. Making things smaller is not the direction folks working with SU(5), SU(10) or E8 are going.

Am I serious about not going to the 15th Eastern Gravity Meeting if the Higgs is found? That is a definitive yes. My family can easily consume the money on more shoes or car repairs. I feel uneasy about my field equations as they would call into question every bit of work done with the Einstein field equations, which is an enormous amount of effort. Go LHC go!

Doug

Snarky puzzle: Write out the Maxwell equations in the Lorenz gauge. Write out the hypercomplex gravity equations in the Lorenz gauge (on the last slide). Subtract the first equations from the second. Shop and compare that with the GEM unified gravity and standard model symmetry field equations written in the last slide. Reflect on how important it is to be similar but not quite the same.

Next late Monday/Tuesday: Cross Dressing Gamma GammaGirls